Long Division

Below is the process written out in full. (It is what is called an "algorithmic process").

You will often see other versions. These are generally just a shortened version

of the process below.

Let's see how it is done with:

425 ÷ 25

There are two definitions we must make first.

the number to be divided into is known as the dividend (425 from above)
The number which divides the other number is known as the divisor (25 from above)

Note: You can also see this done in Long Division Animation

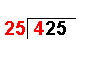	4 ÷ 25 = 0 remainder 4	The first number of the dividend is divided by the divisor.
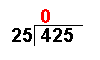		The whole number result is placed at the top. Any remainders are ignored at this point.
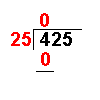	25 × 0 = 0	The answer from the first operation is multiplied by the divisor. The result is placed under the number divided into.
	4 – 0 = 4	Now we subtract the bottom number from the top number.
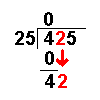		Bring down the next number of the dividend.
	42 ÷ 25 = 1 remainder 17	Divide this number by the divisor.
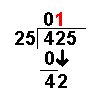		The whole number result is placed at the top. Any remainders are ignored at this point.
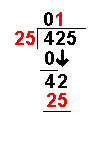	25 × 1 = 25	The answer from the above operation is multiplied by the divisor. The result is placed under the last number divided into.
	42 – 25 = 17	Now we subtract the bottom number from the top number.
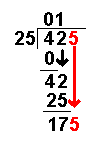		Bring down the next number of the dividend.
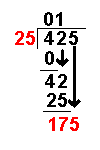	175 ÷ 25 = 7 remainder 0	Divide this number by the divisor.
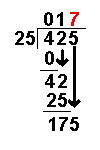		The whole number result is placed at the top. Any remainders are ignored at this point.
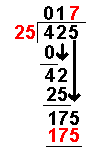	25 × 7 = 175	The answer from the above operation is multiplied by the divisor. The result is placed under the number divided into.
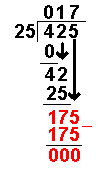	175 – 175 = 0	Now we subtract the bottom number from the top number.
		There are no more numbers to bring down. The answer must be 17

Pages